- Zeitdiskrete dynamische Modellierung geometrisch exakter Balken in absoluten und relativen Variablen. 58. Reglungstechnisches Kolloquium, Boppard, 2024 more…
- Relative-kinematic formulation of geometrically exact beam dynamics based on Lie group variational integrators. Computer Methods in Applied Mechanics and Engineering (Vol. 432, Part A, 117367), 2024 more…
- Efficient Discrete-Time Dynamics of Geometrically Exact Beams Based on Relative Kinematics. 11th ECCOMAS Thematic Conference on Multibody Dynamics, Lissabon, IDMEC - Instituto de Engenharia Mecanica, 2023Multibody2023 more…
- Coordinate-Invariant Modeling and Control of a Three-DOF Robot Manipulator. IFAC-PapersOnLine, Elsevier, 20217th IFAC Workshop on Lagrangian and Hamiltonian Methods for Nonlinear Control, Berlin, pp. 230-236 more…
Geometric Modeling and Control of Flexible Robots
Modern robotic systems can solve highly complex problems with remarkable efficiency, which is often enabled by increasingly complex and partially flexible mechanical structures.
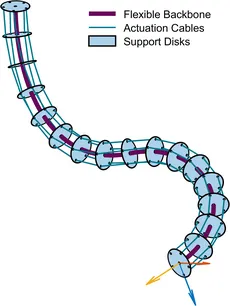
An important example of robots with highly flexible elements are soft robots and continuum manipulators. So-called cable-driven continuum manipulators consist of a highly flexible backbone, which can continuously deform along its entire length and take on complex shapes, and cables (or tendons) routed along the backbone, which are actuated at the base. These robots have (among others) important applications in medical engineering (e.g., for minimally invasive surgery and steerable catheters), in inspection tasks, or manufacturing.
This project focuses on developing structured, numerically efficient methods for modeling, simulation, and model-based control of rigid-flexible robotic systems, such as continuum manipulators.
Geometric modeling of flexible multibody systems
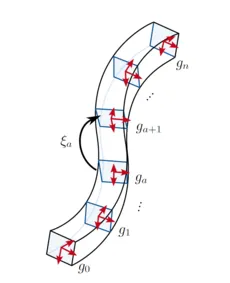
In our modeling approach for multibody systems – i.e., systems of rigid bodies connected with joints or flexible elements --, we aim to preserve and exploit the mathematical structures inherent to such systems. The kinematics of rigid bodies moving in 3D space, consisting of translation and rotation, is naturally described by a Lie group -- the special Euclidean group. This structure can be used to intrinsically consider the complex interaction of rotation and translation elegantly and compactly, resulting in an efficient modeling approach that is physically meaningful, highly structured, and easy to implement in simulation and control tasks.
Highly flexible components are modeled as geometrically exact beams – a general, powerful approach that allows to accurately describe the statics and dynamics of flexible structures undergoing even large deformations. Remarkably, these models have a similar mathematical structure to rigid multibody systems since the configuration of a geometrically exact beam is described by a series of cross-sections along a center line, each behaving like individual rigid bodies in space.
We preserve this Lie group structure under spatial discretization, which is necessary to obtain finite-dimensional models usable for simulation and controller design from the continuous, infinite-dimensional beam models. Preserving the Lie group structure results in high accuracy, even at coarse discretization levels. In the discretized beam, we use relative variables to describe its configuration, which not only results in an efficient minimal model but also simplifies the integration into combined rigid-flexible multibody systems. Very importantly, it also allows us to precisely select which deformation modes (bending, torsion, shear, elongation) should be included in the model in a straightforward way and without using algebraic constraints. This is crucial to achieving good numerical performance since including stiff deformation modes (such as elongation or shear) would require very fine time steps and, thus, high computational effort.
Overall, we work on well-structured, efficient, and general models for tree-structured rigid-flexible multibody systems. The resulting models are implemented in a MATLAB toolbox that will be made publicly available in the future.
Time integration using (Lie group) variational integrators
For accurate yet computationally efficient time integration, we work in the framework of (Lie group) variational integrators. These implicit integrators are based on discrete-time analogs of continuous-time mechanical principles – such as Hamilton’s principle – and result in intrinsically discrete equations of motion, which can preserve the underlying Lie group structure and important physical quantities such as momentum. Moreover, they are symplectic by construction, which gives them excellent long-term energy behavior. Due to this preservation of mathematical and physical structures, variational integrators can achieve accurate results even at large time steps, giving them high numerical efficiency as required in the context of real-time applications.
Model-based (optimal) control of continuum manipulators
One of the primary applications of the developed system models is optimal control, where we aim at efficient and accurate methods that can reliably solve practically relevant problems. Consistent with our discrete-time modeling approach, we solve optimal control problems (OCPs) in the framework of discrete mechanics and optimal control (DMOC), where we discretize the continuous-time control problems using the discrete variational methods outlined above. This directly results in nonlinear programming problems (NLPs) that are easy to implement, computationally efficient, and numerically robust.
Additionally, we investigate observer-based controller structures, e.g., for position or force control of rigid or flexible robot manipulators.
Experimental Systems
For validation, analysis, and testing of the developed models and controller structures, we have two comprehensive experimental robots available at the chair, designed in several student theses.
3R Modular Lightweight Robot Manipulator
This robot serves as a test platform for applications in traditional lightweight robotics and has conventional manipulator kinematics consisting of a shoulder and an elbow joint. The design is fully modular so that different links can be used with varying lengths and stiffness. It is driven by RoboDrive/TQ servo motors with motor and load-sided high-resolution position encoders and high-reduction Harmonic Drive gears.
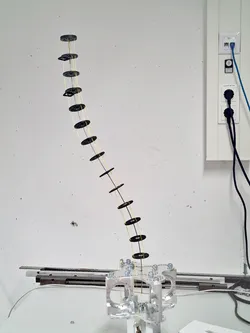
Tendon-driven Continuum Manipulator PETER
PETER (Programmable Elastic Tendon-Excited Robot) is a highly flexible tendon-driven continuum manipulator and, thus, a type of soft robot. It serves as a testing and validation platform for modeling and control approaches for highly flexible systems. While it is primarily built as an experimental platform, it can be used to investigate applications in medical engineering (amongst many others), one of the primary application fields for robots of this type.
PETER is driven by four Sumitomo TUAKA servo motors with integrated controllers and torque sensors. MEMS IMUs (acceleration sensors and gyroscopes) located along the manipulator are used to enable reliable state estimation without any external measurement equipment.
Both robots are controlled by an industrial PLC (from B&R) and programmed with C/C++. This allows for control implementations that are both close to real-world applications and robust and inherently safe. A toolchain based on MATLAB Code generation is used to quickly and efficiently implement complex model-based controllers.