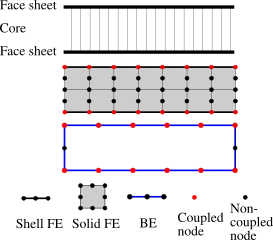
The solution of vibroacoustic problems arising in e.g. automotive and aerospace engineering often requires the simulation of structural acoustic interaction. Typically, the elastodynamic equations underlying the vibrating structure are discretized by the finite element method (FEM), whereas several methods are available for addressing the surrounding and often infinitely large acoustic field. Here, our research mainly focuses on the use of the boundary element method (BEM) and the infinite finite element method (IFEM). We are particularly interested in applications, which involve a mutual, i.e. strong, coupling between the acoustic and the structural responses as it for instance occurs in lightweight structures and musical instruments. In these cases, the fluid loads that act on the structure by virtue of the acoustic field are not negligible.
From a numerical point of view, these fully coupled structural acoustic interaction problems demand a large computational effort, particularly in the case rapidly varying responses over large frequency ranges. In order to address this issue, we employ model order reduction (MOR) techniques. Using MOR involves the computation of a projector, which is suitable to significantly decrease the dimension of the vibroacoustic problem and hence accelerate the subsequent harmonic analysis at different frequency points. The projector can either be precomputed in an offline phase, or updated iteratively during the actual simulations.
Furthermore, we use modal analyses as an alternative approach for studying structural acoustic interactions. Modal analyses require the solution of an often nonlinear eigenvalue problem resulting in structure-inherent properties such as the shifted (so-called wet) eigenfrequencies and the modal radiation loss factors. The latter quantify the extent of structural damping due to sound radiation into the acoustic far-field. Moreover, once the modes are computed, the responses at certain frequencies as well as the responses for different excitations are simply obtained by matrix-vector multiplications.