Discretization Methods: Mortar Methods

Alexander Popp, Philipp Farah and Michael W. Gee

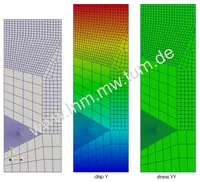
Domain decomposition techniques in general, and mortar methods in particular, provide very powerful tools for the numerical approximation and solution of partial differential equation (PDE) systems. Mortar methods are especially promising when geometrically non-conforming interfaces and / or non-matching triangulations are involved or when different discretization scheme must be coupled. Due to a manifold of reasons (e.g. different resolution requirements in different physical fields, complex geometries) this is most often the case in engineering practice. 

Continuity conditions at the interfaces - usually enforced pointwise via collocation - are satisfied in a weak integral sense with mortar methods, which leads to a variationally consistent mixed formulation based on additional Lagrange multiplier degrees of freedom. The special focus of our research is on so-called dual Lagrange mutliplier spaces, which allow for a much easier realization of the coupling conditions by algebraic condensation of the interface terms. Notably, this approach does not impinge upon the optimality of the method in terms of spatial convergence.

Typical applications at the Institute for Computational Mechanics range from classical domain decomposition (mesh tying) for solid and fluid dynamics to the treatment of surface-coupled problems with non-matching meshes, such as finite deformation contact and fluid-structure interaction.