FlameODE - Flame Response Modeling via Physically Interpretable Differential Equations
by Gregor Doehner and Camilo F. Silva
Motivation
Combustion instabilities remain a challenging obstacle on the road towards ever more efficient and environmentally friendly combustion systems. To predict the occurrence of such instabilities, a flame model, which links relative fluctuations in burner inlet velocity (input) to relative heat release rate fluctuations (output) is generally required. The 'linear' flame response to a given input is traditionally characterized by a finite impulse response (FIR). In the context of numerical simulations, such an FIR model is typically derived by applying broadband forcing to the system's inlet velocity.
To model limit cycles, which are characteristic of unstable thermoacoustic systems, a 'nonlinear' flame response is essential. To date, Wiener-Hammerstein (WH) models are commonly used to characterize such nonlinear behavior. However, these models are typically effective only within a predefined frequency range where the limit cycle is expected to occur. To overcome this limitation, data-driven machine learning (ML) methods, such as Multi-Layer Perceptrons (MLPs) and Long Short-Term Memory (LSTM) networks, have been employed to evaluate the nonlinear flame response over a wide frequency band. While these models are successful, they lack physical interpretability and function as black-box solutions.
Objectives and Strategy
This project aims at utilizing an alternative modeling approach. Here, we aim at describing the flame by a physical substitute system. This system can be described via a parsimonious system of ordinary differential equations (ODEs) that replicates the modeling capabilities of MLPs and LSTMs while remaining compact, especially compared to the thousands of parameters typically required by neural networks. At its current state, the project already demonstrated that a simple mass-spring-damper with nonlinear damping is able to recover key flame dynamics quantitatively in the linear and qualitatively in the nonlinear regime for laminar and turbulent flames. By modifying the architecture to enforce unidirectional information flow (from mass one to mass two only), the model becomes physically interpretable, with each oscillator targeting a specific region of the flame.
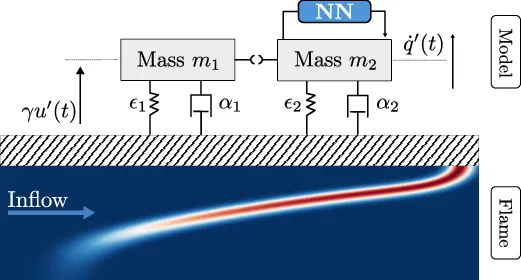
Current & Future Work
Current work focuses on deriving additional nonlinearities which are required to describe the energy transfer between different frequencies (harmonics), an essential feature to adequately describe more complex limit cycles. To do so, we leverage the frameworks of Universal Differential Equations (UDEs) and Sparse Identification of Nonlinear Dynamics (SINDy).
Furthermore, to gain a deeper understanding of flame dynamics, it is essential to understand how parameters of the given mass-spring-damper system relate to the actual flame. Current analysis reveals a strong connection between the base and tip of the flame and mass one and mass two of the substitute ODE system.
Future work will focus on leveraging the ODE system to model more complex flame shapes, different fuels such as hydrogen blends and pure hydrogen, as well as a systematic investigation of nonlinear behavior for different configurations of a simple burning chamber.
If you are a student or researcher and you are interested in collaborating, please contact mailto:gregor.doehner(at)tum.de
Selected References
G. Doehner, Data Driven Modeling of the Laminar Flame Response Using Universal Differential Equations, TU München, München, 2023, http://dx.doi.org/10.13140/RG.2.2.30348.49285
Gregor Doehner, Alexander J. Eder, Camilo F. Silva, A parsimonious system of ordinary differential equations for the response modeling of turbulent swirled flames, Combustion and Flame, 2024, http://dx.doi.org/10.1016/j.combustflame.2024.113408
Doehner G, Haeringer M, Silva CF. Nonlinear flame response modelling by a parsimonious set of ordinary differential equations, International Journal of Spray and Combustion Dynamics, 2022, http://dx.doi.org/10.1177/17568277221094760